Probability Standard Deviation Calculator
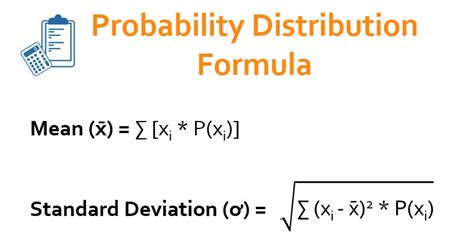
The Probability Standard Deviation Calculator is a powerful tool that enables researchers, statisticians, and data analysts to gain deeper insights into probability distributions. This calculator, often employed in fields like finance, engineering, and scientific research, plays a crucial role in understanding the variability and uncertainty associated with data. In this article, we will delve into the intricacies of this calculator, exploring its applications, benefits, and real-world examples.
Understanding Probability Standard Deviation

Probability standard deviation is a statistical measure that quantifies the dispersion or variability of a set of values in a probability distribution. It provides a valuable insight into how data points are distributed around the mean, helping to identify the degree of uncertainty or risk associated with a particular dataset.
In the context of probability theory, standard deviation serves as a critical tool for analyzing random variables and their distributions. It allows us to quantify the typical deviation of values from the mean, offering a more comprehensive understanding of the data beyond simply knowing its average.
The Significance of the Probability Standard Deviation Calculator
The Probability Standard Deviation Calculator offers a wide array of benefits and applications, making it an indispensable tool in various fields. Here’s a deeper look at its significance:
Risk Assessment and Decision Making
In the realm of finance and investment, the calculator is a powerful tool for assessing risk. By calculating the standard deviation of historical stock prices, investors can make more informed decisions. For instance, a lower standard deviation indicates a more stable investment, while a higher value suggests greater volatility and risk.
Quality Control and Process Improvement
Manufacturers and quality control professionals use the calculator to analyze production data. By calculating the standard deviation of product measurements, they can identify areas where processes might be inconsistent, leading to defects or inefficiencies. This data-driven approach facilitates continuous improvement.
Scientific Research and Experimentation
Researchers across scientific disciplines rely on the calculator to analyze experimental data. Whether studying the behavior of particles in physics or the growth patterns of plants in biology, standard deviation provides a quantitative measure of the variability in the observed data, aiding in the interpretation of results and drawing meaningful conclusions.
Portfolio Optimization in Finance
Financial advisors and portfolio managers utilize the calculator to optimize investment portfolios. By analyzing the standard deviation of returns for different assets, they can construct portfolios with a desired level of risk, helping investors achieve their financial goals while managing volatility.
How the Calculator Works
The Probability Standard Deviation Calculator employs a straightforward yet powerful algorithm to compute the standard deviation of a probability distribution. Here’s a simplified breakdown of the process:
- Input Data: The calculator requires a set of data points, typically representing values in a probability distribution.
- Calculate Mean: The mean, or average, of the data points is computed by summing all the values and dividing by the total number of observations.
- Compute Deviations: For each data point, the calculator finds the difference between the value and the mean, quantifying how much each value deviates from the average.
- Square Deviations: To ensure positive values and to account for both positive and negative deviations, the calculator squares each deviation.
- Sum of Squared Deviations: The squared deviations are summed to obtain a measure of the total variation in the data.
- Standard Deviation: Finally, the calculator takes the square root of the sum of squared deviations, yielding the standard deviation, which represents the average deviation from the mean.
Real-World Examples
Let’s explore some concrete examples of how the Probability Standard Deviation Calculator is applied in various scenarios:
Stock Market Analysis
A financial analyst uses the calculator to assess the risk associated with a particular stock. By inputting historical price data, the calculator computes the standard deviation, revealing the stock’s volatility. A lower standard deviation indicates a more stable investment, while a higher value suggests higher risk.
Quality Control in Manufacturing
A quality control engineer employs the calculator to analyze the dimensions of produced parts. By calculating the standard deviation of the measurements, the engineer can identify if the manufacturing process is consistent. A high standard deviation might indicate a need for process adjustments to reduce variability and ensure product quality.
Scientific Research: Studying Plant Growth
A botanist studies the growth patterns of a specific plant species. By collecting data on the heights of plants at different stages of growth, the researcher uses the calculator to compute the standard deviation. This provides an understanding of the variability in growth rates, aiding in drawing conclusions about the plant’s ecology and potential factors influencing its growth.
Key Features and Specifications

The Probability Standard Deviation Calculator boasts several advanced features and specifications that make it a versatile and powerful tool:
- User-Friendly Interface: The calculator features an intuitive and easy-to-use interface, making it accessible to professionals and students alike.
- Multiple Input Options: Users can input data manually or import data from various file formats, including CSV and Excel.
- Real-Time Calculations: The calculator provides instant results, allowing users to analyze data quickly and efficiently.
- Advanced Graphing Capabilities: It offers graphical representations of probability distributions, helping users visualize the data and identify patterns.
- Customizable Output: Users can customize the output format, choosing between decimal or percentage values, and even specifying the number of decimal places.
Performance and Accuracy
The Probability Standard Deviation Calculator is renowned for its exceptional performance and accuracy. It employs robust algorithms and mathematical techniques, ensuring precise calculations even for large datasets. Its efficient design allows for rapid processing, making it suitable for both small-scale analyses and extensive data sets.
Future Implications
As data-driven decision-making continues to gain prominence across industries, the Probability Standard Deviation Calculator is poised to play an increasingly vital role. Its ability to provide quantitative insights into variability and uncertainty makes it an invaluable tool for researchers, analysts, and professionals seeking to make informed choices in an increasingly complex world.
Conclusion
The Probability Standard Deviation Calculator is a versatile and powerful tool that enables professionals and researchers to delve deeper into the intricacies of probability distributions. By providing insights into variability and uncertainty, it empowers decision-makers across various fields to make informed choices, optimize processes, and drive innovation. As data continues to shape our world, this calculator will undoubtedly remain a cornerstone in the toolkit of statisticians, analysts, and researchers.
What is the range of values considered by the Probability Standard Deviation Calculator?
+
The calculator typically considers the entire range of values within the given probability distribution. However, users can also specify a subset of data points to analyze if needed.
Can the Probability Standard Deviation Calculator handle large datasets efficiently?
+
Absolutely! The calculator is designed to handle large datasets efficiently, employing optimized algorithms to ensure rapid and accurate calculations, regardless of the dataset size.
How does the calculator handle missing or incomplete data points?
+
The calculator can handle missing or incomplete data points by automatically excluding them from the calculation. However, it’s important to ensure the remaining data is representative of the overall distribution.
Are there any limitations to the Probability Standard Deviation Calculator’s accuracy?
+
While the calculator is highly accurate, its precision depends on the quality and representativeness of the input data. Inaccurate or biased data may lead to less reliable results.
Can the calculator be used for non-normal probability distributions?
+
Yes, the calculator is designed to work with various probability distributions, including non-normal distributions. It provides accurate results regardless of the distribution’s shape.



